На русском языке
Gravitational
four-potential
Gravitational four-potential is a four-vector function (4-vector), by
which the properties of gravitational field are determined in the Lorentz-invariant theory of gravitation, [1] as well as in the covariant theory of gravitation. [2] The gravitational 4-potential includes the scalar
and vector potentials of gravitational field. During the gauge transformation
the potentials of the gravitational field can change their form so that
non-coinciding 4-potentials with different dependence on the coordinates and
time can correspond to the same gravitational field.
Contents
|
Definition
According to the metric theory of relativity, the same physical phenomena can be recorded and described not only by
electromagnetic waves, but also by other waves, such as gravitational waves. In
the gravitational-wave representation, the speed of gravitation ![]() should be used instead of the
speed of light
should be used instead of the
speed of light ![]() .
.
In this case gravitational
4-potential, like any 4-vector, consists of the scalar and vector parts, which
in sum give 4 components:
![]()
The time-like component of the 4-potential is the scalar
potential ![]() , divided by
, divided by ![]() . The space-like component of the
4-potential is represented by the vector potential of gravitational field
. The space-like component of the
4-potential is represented by the vector potential of gravitational field ![]() , which has three components.
, which has three components.
Since several fields act
simultaneously in real phenomena, it is convenient to use the
electromagnetic-wave representation for all these fields, which allows us to
compare the action of the fields without recalculating the units of measurement
of physical quantities. In the electromagnetic-wave representation, the scalar
and vector potentials are defined so that the gravitational 4-potential has the
following form:
![]()
Definition (1) of the 4-potential ![]() in
the covariant representation with a lower index is preferred to the
contravariant representation (with an upper index), as it makes the solution of
equations easier.
in
the covariant representation with a lower index is preferred to the
contravariant representation (with an upper index), as it makes the solution of
equations easier.
In the transition from one reference frame to another the
4-potential is transformed in accordance with the axioms of the metric theory of relativity.
In case of Minkowski space of the special theory of relativity transformations
of the 4-potential are performed from one inertial frame to another using
Lorentz transformations.
In the international system of units SI the gravitational
4-potential ![]() is
measured in m/s, in the system of physical units CGS – in cm/s.
is
measured in m/s, in the system of physical units CGS – in cm/s.
Relation with gravitational field
strength and torsion field
By the gravitational 4-potential (1) the gravitational tensor is determined, for
this purpose a 4-rotor is used:
![]()
The antisymmetric tensor
![]() contains only 6 components, three
of which are associated with the vector of gravitational
field strength
contains only 6 components, three
of which are associated with the vector of gravitational
field strength ![]() , and the other three components – with the vector of the gravitational torsion field
, and the other three components – with the vector of the gravitational torsion field ![]() . In Cartesian coordinates these vectors
are obtained as follows:
. In Cartesian coordinates these vectors
are obtained as follows:
![]()
![]()
From the latter relation we see that the torsion field
depends only on the vector potential. At the same time, contribution into the
gravitational field strength is made not only by the gradient of the scalar
potential, but also by the rate of change in time of the vector potential.
Gauge fixing of 4-potential
The most
convenient is the gauge, when the 4-divergence of the 4-potential is zero:
![]()
In
the special relativity the covariant derivative ![]() becomes a
partial derivative
becomes a
partial derivative ![]() . This allows us,
taking
into account (1), to represent the gauge condition explicitly as follows:
. This allows us,
taking
into account (1), to represent the gauge condition explicitly as follows:
![]()
In the
Lorentz-invariant theory of gravitation the Heaviside equations for
gravitational field are written in the four-dimensional form::
![]()
![]()
It can be shown
that the gauge condition of the 4-potential (3) follows from the definition of
the gravitational tensor (2) and the field equations (4) and (5). If we apply
the partial derivative ![]() to (5),
then its action in the first two terms in (5) on the tensor
to (5),
then its action in the first two terms in (5) on the tensor ![]() can be
considered with the help of (4). For the third term in (5) we obtain the
relation
can be
considered with the help of (4). For the third term in (5) we obtain the
relation ![]() , where
, where ![]() is the 4-d'Alembertian operator:
is the 4-d'Alembertian operator:
![]()
here
the Laplace operator is applied, which in Cartesian coordinates has
the form ![]()
Substituting in
(5) ![]() with its
expression according to (2), from (5) as
a simplest option we obtain the wave equation for the 4-potential, the source of which is the mass
4-current
with its
expression according to (2), from (5) as
a simplest option we obtain the wave equation for the 4-potential, the source of which is the mass
4-current ![]() : [3]
: [3]
![]()
where ![]() is
the gravitational constant.
is
the gravitational constant.
On the other hand, if in (4) we substitute ![]() with its
expression according to (2), we obtain again the wave equation (6) for the 4-potential,
but only given the gauge conditions of the 4-potential (3) is met. Thus, due to the symmetry of
fields, this calibration can simplify the field equations.
with its
expression according to (2), we obtain again the wave equation (6) for the 4-potential,
but only given the gauge conditions of the 4-potential (3) is met. Thus, due to the symmetry of
fields, this calibration can simplify the field equations.
Note that if we
take the partial derivative ![]() of both
sides in (4), then taking into account (2) the left side is equal to zero. Then
from the equality of the right side to zero the continuity
equation follows for the mass 4-current:
of both
sides in (4), then taking into account (2) the left side is equal to zero. Then
from the equality of the right side to zero the continuity
equation follows for the mass 4-current:
![]()
If we subtract
from the gravitational 4-potential ![]() the gauge
4-vector of the form
the gauge
4-vector of the form ![]() , depending on a
scalar gauge function
, depending on a
scalar gauge function ![]() , then provided
that the function
, then provided
that the function ![]() satisfies
the wave equation
satisfies
the wave equation
![]()
for the new
4-potential ![]() the gauge condition (3) will remain in force,
and the gravitational tensor according to (2) will not change its form. Thus,
the Lorentz-invariant theory of gravitation and built upon it the covariant
theory of gravitation are gauge theories.
the gauge condition (3) will remain in force,
and the gravitational tensor according to (2) will not change its form. Thus,
the Lorentz-invariant theory of gravitation and built upon it the covariant
theory of gravitation are gauge theories.
In the covariant theory of
gravitation the Heaviside equations (4) and (5) for gravitational field are
generalized for the curved spacetime and are written in such form: [2]
] [4] [5]
![]()
![]()
Wave equation
instead of (6) is as follows:
![]()
In the curved
spacetime in the equation we should take into account mixing of the vector
components. In particular, the scalar potential of gravitational field becomes
a function not only of the mass density ![]() of moving
matter, but also of the
mass current density
of moving
matter, but also of the
mass current density ![]() , where
, where ![]() is the
velocity of the matter.
is the
velocity of the matter.
Solution of wave equation for
4-potential
In the special theory
of relativity, the Christoffel symbols ![]() are zero, and then the solution of the wave equation can be simplified
so that it can be represented as follows: [2]
are zero, and then the solution of the wave equation can be simplified
so that it can be represented as follows: [2]
![]()
where the gravitational 4-potential ![]() at
the time point
at
the time point ![]() at the point of the space determined by the radius vector
at the point of the space determined by the radius vector ![]() , is found by integrating over the volume,
containing the mass 4-current (or 4-vector of mass current density)
, is found by integrating over the volume,
containing the mass 4-current (or 4-vector of mass current density) ![]() . In this case integration over the volume
is carried out for an earlier point in time
. In this case integration over the volume
is carried out for an earlier point in time ![]() , where
, where ![]() is
the radius vector that specifies the location of the mass 4-current at the
earlier time.
is
the radius vector that specifies the location of the mass 4-current at the
earlier time.
From the given solution
for the time-like components of 4-vectors we can see that the scalar potential
depends on the mass density of a certain moving particle at the earlier point
in time and on the distance from this particle to the point where the potential
is measured. In turn, the vector potential also depends on the speed of the
particle at the earlier point in time. The presence of the volume integral
implies that for the potentials of gravitational field the superposition
principle holds, and for calculating the total 4-potential we should take into
account all sources of the field.
The 4-potential of the proper gravitational field of a single solid point particle can be obtained in another way – by
multiplying the scalar gravitational potential
![]() around this particle, calculated in the
reference frame co-moving with this particle, by the 4-velocity of the point
particle:
around this particle, calculated in the
reference frame co-moving with this particle, by the 4-velocity of the point
particle:

For the observer, relative to which the point particle is
moving, according to the Lorentz transformations the scalar potential changes
due to the motion of the particle: 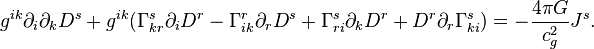 , and
also the vector potential appears, which is equal to
, and
also the vector potential appears, which is equal to 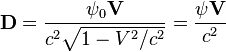 .
This gives the ordinary definition of the gravitational 4-potential in
the form
.
This gives the ordinary definition of the gravitational 4-potential in
the form
![]()
Indeed, the 4-potential of any vector field for a
single particle, inside which the vector potentials of the fields are absent,
can be represented as follows: [6] [7]
![]()
where ![]() for electromagnetic field and
for electromagnetic field and ![]() for other fields,
for other fields, ![]() and
and ![]() are the mass density and
accordingly charge density in comoving reference frame,
are the mass density and
accordingly charge density in comoving reference frame, ![]() is the field energy density of
the particle,
is the field energy density of
the particle, ![]() is the covariant four-velocity.
is the covariant four-velocity.
For
gravitational field there is ![]() ,
,
![]() , and we arrive to formula for the 4
potential
, and we arrive to formula for the 4
potential ![]() .
.
In a system of a set of point particles composing
material bodies, in order to find the total 4-potential we should add
4-potentials of all point particles, taking into account the differences in
their 4-velocities and their different location in space. As a result the total
vector potential of the system of particles only indirectly reflects the total
scalar potential of the given system of particles, in contrast to the direct
relation between the scalar and vector potentials of an individual point particle.
In the case of calculating the total 4-potential of a massive solid body, given
the different distances from the parts of the body to the point where the
4-potential is defined, we obtain the gravitational Liénard–Wiechert
potential. [8] [9]
The gravitational 4-potential for relativistic uniform system is calculated
in the paper. [10]
Lagrangian and action
The
gravitational 4-potential is part of the Lagrangian for the matter in
gravitational and electromagnetic fields that allows us to write the
corresponding action function: [11] [4]
![]()
![]()
where ![]() is Lagrangian,
is Lagrangian, ![]() is the time differential of the
reference frame used,
is the time differential of the
reference frame used, ![]() is a certain coefficient,
is a certain coefficient, ![]() is the scalar curvature,
is the scalar curvature, ![]() is the cosmological constant, which
characterizes the energy density of the considered system as a whole and
therefore is the function of the system,
is the cosmological constant, which
characterizes the energy density of the considered system as a whole and
therefore is the function of the system, ![]() is the speed of light as a
measure of the propagation speed of electromagnetic and gravitational
interactions, the electromagnetic 4-potential
is the speed of light as a
measure of the propagation speed of electromagnetic and gravitational
interactions, the electromagnetic 4-potential
![]() where
where ![]() is the scalar potential, and
is the scalar potential, and ![]() is the vector potential,
is the vector potential, ![]() – electric four-current,
– electric four-current, ![]() is the electric constant,
is the electric constant, ![]() – electromagnetic tensor,
– electromagnetic tensor, ![]() – acceleration tensor,
– acceleration tensor, ![]() and
and ![]() are some constants,
are some constants, ![]() is four-potential of pressure field,
is four-potential of pressure field, ![]() –
pressure field tensor,
–
pressure field tensor, ![]() is
the invariant 4-volume expressed through the differential of the time
coordinate
is
the invariant 4-volume expressed through the differential of the time
coordinate ![]() , through the product
, through the product ![]() of differentials of the space
coordinates, and through the square root
of differentials of the space
coordinates, and through the square root ![]() of the determinant
of the determinant ![]() of the metric tensor taken with a
negative sign.
of the metric tensor taken with a
negative sign.
In the action integral the gravitational 4-potential is
present within the invariant ![]() , and as well as part of the gravitational
field tensor
, and as well as part of the gravitational
field tensor ![]() and its invariant
and its invariant ![]() . In the first case, the 4-potential
determines the function of the binding energy of the substance and the field,
and in the second case it determines the energy function of the field as an
independent object. The variation of the action function leads to the
determination of the gravitational
stress-energy tensor of the gravitational field, sets the gravitational
field equations (7) and (8), the equation of the substance motion in the field
and the expression for the gravitational four-force.
. In the first case, the 4-potential
determines the function of the binding energy of the substance and the field,
and in the second case it determines the energy function of the field as an
independent object. The variation of the action function leads to the
determination of the gravitational
stress-energy tensor of the gravitational field, sets the gravitational
field equations (7) and (8), the equation of the substance motion in the field
and the expression for the gravitational four-force.
Role of 4-potential in theory of
gravitation
In classical mechanics instead of the total 4-potential
its scalar component is used in the form of the gravitational potential. This
allows us to find the potential gravitational energy of bodies and the
equations of their motion. In order to calculate the scalar gravitational
potential the Poisson equation is used of the form: ![]() , where
, where ![]() is
the Laplace operator,
is
the Laplace operator, ![]() is
the volume density of the mass distribution at the considered point. However,
the expressions obtained for the potential, forces and energies are not Lorentz
covariant, that is, the problem arises during the translation of the results
from one inertial frame to another.
is
the volume density of the mass distribution at the considered point. However,
the expressions obtained for the potential, forces and energies are not Lorentz
covariant, that is, the problem arises during the translation of the results
from one inertial frame to another.
The gravitational 4-potential practically is not
considered in the general relativity (GR). This is due to the fact that in GR
the gravitational field is considered identical to the metric field, and the
components of the metric tensor are used as the gravitational potentials, and
the Christoffel symbols are used instead of the field strength. In the weak
field the relation can be established between the component ![]() of
the metric tensor of the spacetime and the gravitational scalar potential in
classical mechanics:
of
the metric tensor of the spacetime and the gravitational scalar potential in
classical mechanics: ![]() , where
, where ![]() is
the speed of light. The vector potential of gravitational field
is
the speed of light. The vector potential of gravitational field ![]() that is used in the Lorentz-invariant theory
of gravitation can also be expressed in terms of the components of the metric
tensor of GR.
that is used in the Lorentz-invariant theory
of gravitation can also be expressed in terms of the components of the metric
tensor of GR.
On the other hand, in the axiomatic construction of the Lorentz-invariant theory of gravitation
(LITG) it is the 4-potential ![]() that represents the gravitational field, while
as for the substance it is represented by the mass 4-current
that represents the gravitational field, while
as for the substance it is represented by the mass 4-current ![]() . The fifth axiom of LITG states that
d'Alembertian of the 4-potential equals the mass 4-current with a corresponding
constant factor. [2] It is sufficient to derive all of the
relations of the Lorentz-invariant theory of gravitation. The axiomatics of
LITG is the same for the covariant theory
of gravitation (CTG), since CTG is the generalization of LITG to the curved
spacetime, in which the metric tensor is dependent on the time and coordinates.
. The fifth axiom of LITG states that
d'Alembertian of the 4-potential equals the mass 4-current with a corresponding
constant factor. [2] It is sufficient to derive all of the
relations of the Lorentz-invariant theory of gravitation. The axiomatics of
LITG is the same for the covariant theory
of gravitation (CTG), since CTG is the generalization of LITG to the curved
spacetime, in which the metric tensor is dependent on the time and coordinates.
The gravitational 4-potential as well as the
electromagnetic 4-potential, acting on test bodies, influences the rate of time
in these bodies. [12] This leads to the fact that
the same processes that occur in bodies, which are located in different
4-potentials, get out of phase. For the phase shift between two identical
particles with the mass ![]() and the charge
and the charge ![]() , one of which is in a certain
gravitational (electromagnetic) field, we obtain:
, one of which is in a certain
gravitational (electromagnetic) field, we obtain:

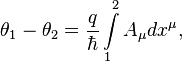
here ![]() is
the Dirac constant,
is
the Dirac constant, ![]() is the electromagnetic 4-potential,
is the electromagnetic 4-potential, ![]() is
the 4-displacement of the particle in space and time.
is
the 4-displacement of the particle in space and time.
The latter relation for the phase shift in
electromagnetic field is confirmed by the Aharonov-Bohm effect.
Using the gravitational 4-potential, the generalized
4-momentum is calculated, [13] which is part of the
4-momentum of a physical system of particles and fields. [14]
The field
energy theorem, which has the same meaning for fields as
the virial theorem for particles, is applicable to a vector gravitational field
in a curved spacetime. In the formulation of the theorem there is the
gravitational 4-potential: [15]

See also
- Gravitational potential
- Gravitational vector potential
- Electromagnetic four-potential
References
- Fedosin S.G. Fizika i filosofiia podobiia ot preonov do metagalaktik,
Perm, pages 544, 1999. ISBN 5-8131-0012-1.
- 2.0 2.1 2.2 2.3 Fedosin S.G. Fizicheskie teorii i beskonechnaia
vlozhennost’ materii.
– Perm, 2009, 844 pages, Tabl. 21, Pic. 41, Ref.
289. ISBN 978-5-9901951-1-0. (in Russian).
- Fedosin S.G. Sovremennye problemy fiziki: v poiskakh novykh printsipov.
Moskva: Editorial URSS, 2002, 192 pages. ISBN 5-8360-0435-8.
- 4.0 4.1 Fedosin
S.G. About the cosmological
constant, acceleration field, pressure field and energy.
Jordan Journal of Physics. Vol. 9, No. 1, pp. 1-30 (2016). http://dx.doi.org/10.5281/zenodo.889304.
- Fedosin S.G.
Lagrangian formalism in the theory of relativistic vector fields.
International Journal of Modern Physics A, Vol. 40, No. 02, 2450163 (2025). https://doi.org/10.1142/S0217751X2450163.
- Fedosin S.G. The
procedure of finding the stress-energy tensor and vector field equations
of any form. Advanced Studies in Theoretical Physics, Vol. 8, no. 18, pp. 771-779 (2014). http://dx.doi.org/10.12988/astp.2014.47101.
- Fedosin S.G. Equations of Motion in the Theory of Relativistic
Vector Fields. International Letters of Chemistry, Physics and Astronomy,
Vol. 83, pp. 12-30 (2019). https://doi.org/10.18052/www.scipress.com/ILCPA.83.12.
- Fedosin S.G. Energy, Momentum, Mass and Velocity of a Moving Body. Preprints 2017, 2017040150. http://dx.doi.org/10.20944/preprints201704.0150.v1.
- Fedosin S.G. Energy, Momentum, Mass and
Velocity of a Moving Body in the Light of Gravitomagnetic Theory.
Canadian Journal of Physics, Vol. 92, No. 10, pp. 1074-1081 (2014). http://dx.doi.org/10.1139/cjp-2013-0683.
- Fedosin S.G. The Gravitational
Field in the Relativistic Uniform Model within the Framework of the
Covariant Theory of Gravitation. International Letters of Chemistry,
Physics and Astronomy, Vol. 78, pp. 39-50 (2018). http://dx.doi.org/10.18052/www.scipress.com/ILCPA.78.39. // Гравитационное поле в релятивистской однородной модели в рамках ковариантной теории гравитации,
- Fedosin S.G. The Principle of Least Action in Covariant Theory of Gravitation.
Hadronic Journal, Vol. 35, No. 1, pp. 35-70 (2012). http://dx.doi.org/10.5281/zenodo.889804.
- Fedosin S.G. The Hamiltonian in Covariant Theory of Gravitation. Advances in Natural Science, Vol. 5, No. 4, pp.
55-75 (2012). http://dx.doi.org/10.3968%2Fj.ans.1715787020120504.2023.
- Fedosin S.G.
Generalized Four-momentum for Continuously Distributed Materials. Gazi
University Journal of Science, Vol. 37, Issue 3, pp. 1509-1538
(2024). https://doi.org/10.35378/gujs.1231793. // Обобщённый 4-импульс для непрерывно распределённого вещества,
- Fedosin S.G. What should
we understand by the four-momentum of physical system? Physica Scripta, Vol. 99, No. 5, 055034 (2024). https://doi.org/10.1088/1402-4896/ad3b45.
// Что
мы должны понимать под 4-импульсом физической системы?
- Fedosin S.G. The Integral Theorem of the Field Energy.
Gazi University Journal of Science. Vol. 32, No. 2, pp. 686-703 (2019). http://dx.doi.org/10.5281/zenodo.3252783.