Dissipation field tensor

The dissipation field tensor is an antisymmetric tensor describing the energy dissipation due to viscosity and consisting of six components. Tensor components are at the same time components of the two three-dimensional vectors – dissipation field strength and the solenoidal dissipation vector. With the dissipation field tensor the dissipation stress-energy tensor, the dissipation field equations and dissipation force in matter are defined. Dissipation field is a component of general field.

1 Definition
2 Expression for the components
3 Properties of tensor
4 Dissipation field
5 Covariant theory of gravitation

5.1 Action and Lagrangian
5.2 Dissipation stress-energy tensor
5.3 Generalized velocity and Hamiltonian

6 See also
7 References
8 External links

Definition

Expression for the dissipation field tensor can be found in papers by Sergey Fedosin, ^[1] where the tensor is defined using 4-curl:

$h_{\mu \nu} = \nabla_\mu \lambda_\nu - \nabla_\nu \lambda_\mu = \frac{\partial \lambda_\nu}{\partial x^\mu} - \frac{\partial \lambda_\mu}{\partial x^\nu}.\qquad\qquad (1)$

Here dissipation 4-potential $~ \lambda_\mu$ is given by:

$~\lambda_\mu = \left( \frac {\varepsilon }{ c}, -\mathbf{\Theta } \right),$

where $~\varepsilon$ is the scalar potential, $~ \mathbf{\Theta }$ is the vector potential of dissipation field, – speed of light.

Expression for the components

The dissipation field strength and the solenoidal dissipation vector are found with the help of (1):

$~ X_i= c (\partial_0 \lambda_i -\partial_i \lambda_0),$

$~ Y_k= \partial_i \lambda_j -\partial_j \lambda_i ,$

and the same in vector notation:

$~\mathbf{X}= -\nabla \varepsilon - \frac{\partial \mathbf{\Theta}} {\partial t},$

$~\mathbf{Y }= \nabla \times \mathbf{\Theta }.$

The dissipation field tensor consists of the components of these vectors:

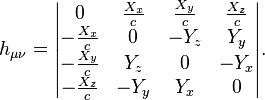
$~ h_{\mu \nu}= \begin{vmatrix} 0 & \frac {X_x}{ c} & \frac {X_y}{ c} & \frac {X_z}{ c} \\ -\frac {X_x}{ c} & 0 & - Y_{z} & Y_{y} \\ -\frac {X_y}{ c} & Y_{z} & 0 & -Y_{x} \\ -\frac {X_z}{ c}& -Y_{y} & Y_{x} & 0 \end{vmatrix}.$

The transition to the dissipation field tensor with contravariant indices is carried out by multiplying by double metric tensor:

$~ h^{\alpha \beta}= g^{\alpha \nu} g^{\mu \beta} h_{\mu \nu}.$

In the special relativity, this tensor has the form:

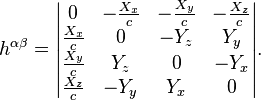
$~ h^{\alpha \beta}= \begin{vmatrix} 0 &- \frac {X_{x}}{ c} & -\frac {X_{y}}{ c} & -\frac {X_{z}}{ c} \\ \frac {X_{x}}{ c} & 0 & - Y_{z} & Y_{y} \\ \frac {X_{y}}{ c}& Y_{z} & 0 & -Y_{x} \\ \frac {X_{z}}{ c}& -Y_{y} & Y_{x} & 0 \end{vmatrix}.$

To convert the components of the dissipation field tensor from one inertial system to another we must take into account the transformation rule for tensors. If the reference frame K' moves with an arbitrary constant velocity $~ \mathbf {V}$ with respect to the fixed reference system K, and the axes of the coordinate systems are parallel to each other, the dissipation field strength and the solenoidal dissipation vector are converted as follows:

$\mathbf {X}^\prime = \frac {\mathbf {V}}{V^2} (\mathbf {V}\cdot \mathbf {X}) + \frac {1}{\sqrt{1 - {V^2 \over c^2}}} \left(\mathbf {X}-\frac {\mathbf {V}}{V^2} (\mathbf {V}\cdot \mathbf {X}) + [\mathbf {V} \times \mathbf {Y }] \right),$

$\mathbf {Y }^\prime = \frac {\mathbf {V}}{V^2} (\mathbf {V}\cdot \mathbf {Y }) + \frac {1}{\sqrt{1 - {V^2 \over c^2}}} \left(\mathbf {Y }-\frac {\mathbf {V}}{V^2} (\mathbf {V}\cdot \mathbf {Y }) - \frac {1}{ c^2} [\mathbf {V} \times \mathbf {X}] \right).$

Properties of tensor

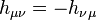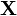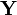$~ h_{\mu \nu}$ is the antisymmetric tensor of rank 2, from this the condition follows $~ h_{\mu \nu} =-h_{\nu \mu}$ . Three of the six independent components of the dissipation field tensor are associated with the components of the dissipation field strength $~ \mathbf {X}$ , and the other three – with the components of the solenoidal dissipation vector $~ \mathbf {Y}$ . Due to the antisymmetry such invariant as the contraction of the tensor with the metric tensor vanishes: $~ g^{\mu \nu} h_{\mu \nu}= h^{\mu}_\mu =0$ .
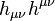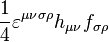Contraction of tensor with itself $h_{\mu \nu} h^{\mu \nu}$ is an invariant, and the contraction of tensor product with Levi-Civita symbol as $\frac {1}{4} \varepsilon^{\mu \nu \sigma \rho} h_{\mu \nu} f_{\sigma \rho}$ is the pseudoscalar invariant. These invariants in the special relativity can be expressed as follows:

$h_{\mu \nu} h^{\mu \nu} = -\frac {2}{c^2} (X^2- c^2 Y^2) = inv,$

$\frac {1}{4} \varepsilon^{\mu \nu \sigma \rho}h_{\mu \nu} h_{\sigma \rho} = - \frac {2}{ c } \left( \mathbf X \cdot \mathbf {Y} \right) = inv.$

Determinant of the tensor is also Lorentz invariant:

$\det \left( h_{\mu \nu} \right) = \frac{4}{c^2} \left(\mathbf X \cdot \mathbf {Y} \right)^{2}.$

Dissipation field

Through the dissipation field tensor the equations of dissipation field are written:

$\nabla_\sigma h_{\mu \nu}+\nabla_\mu h_{\nu \sigma}+\nabla_\nu h_{\sigma \mu}=\frac{\partial h_{\mu \nu}}{\partial x^\sigma} + \frac{\partial h_{\nu \sigma}}{\partial x^\mu} + \frac{\partial h_{\sigma \mu}}{\partial x^\nu} = 0. \qquad\qquad (2)$

$~ \nabla_\nu h^{\mu \nu} = - \frac{4 \pi \tau }{c^2} J^\mu, \qquad\qquad (3)$

where $J^\mu = \rho_{0} u^\mu$ is the mass 4-current, $\rho_{0}$ is the mass density in comoving reference frame, $u^\mu$ is the 4-velocity, $~ \tau$ is a constant.

Instead of (2) it is possible to use the expression:

$~ \varepsilon^{\mu \nu \sigma \rho}\frac{\partial h_{\mu \nu}}{\partial x^\sigma} = 0 .$

Equation (2) is satisfied identically, which is proved by substituting into it the definition for the dissipation field tensor according to (1). If in (2) we insert tensor components $h_{\mu \nu}$ , this leads to two vector equations:

$~ \nabla \times \mathbf{X} = - \frac{\partial \mathbf{Y} } {\partial t} , \qquad\qquad (4)$

$~ \nabla \cdot \mathbf{Y} = 0 . \qquad\qquad (5)$

According to (5), the solenoidal dissipation vector has no sources as its divergence vanishes. From (4) it follows that the time variation of the solenoidal dissipation vector leads to a curl of the dissipation field strength.

Equation (3) relates the dissipation field to its source in the form of mass 4-current. In Minkowski space of special relativity the form of the equation is simplified and becomes:

$~ \nabla \cdot \mathbf{X} = 4 \pi \tau \rho,$

$~ \nabla \times \mathbf{Y} = \frac{1}{c^2} \left( 4 \pi \tau \mathbf{J} + \frac{\partial \mathbf{X}} {\partial t} \right),$

где $~ \rho$ – плотность движущейся массы, $~ \mathbf{J}$ – плотность тока массы.

According to the first of these equations, the dissipation field strength is generated by the mass density, and according to the second equation the mass current or change in time of the dissipation field strength generate the circular field of the solenoidal dissipation vector.

From (3) and (1) it can be obtained: ^[2]

$~ R_{ \mu \alpha } h^{\mu \alpha }= \frac {4 \pi \tau }{c^2} \nabla_{\alpha}J^{\alpha}.$

The continuity equation for the mass 4-current $~\nabla _{{\alpha }}J^{{\alpha }}=0$ is a gauge condition that is used to derive the field equation (3) from the principle of least action. Therefore, the contraction of the dissipation field tensor and the Ricci tensor must be zero: $~R_{{\mu \alpha }}h^{{\mu \alpha }}=0$ . In Minkowski space the Ricci tensor $~R_{{\mu \alpha }}$ equal to zero, the covariant derivative becomes the partial derivative, and the continuity equation becomes as follows:

$~\partial_{\alpha } J^\alpha = \frac {\partial \rho } {\partial t}+ \nabla \cdot \mathbf{J} =0.$

Covariant theory of gravitation

Action and Lagrangian

Total Lagrangian for the matter in gravitational and electromagnetic fields includes the dissipation field tensor and is contained in the action function: ^[1]

$~S =\int {L dt}=\int (kR-2k \Lambda - \frac {1}{c}D_\mu J^\mu + \frac {c}{16 \pi G} \Phi_{ \mu\nu}\Phi^{ \mu\nu} -\frac {1}{c}A_\mu j^\mu - \frac {c \varepsilon_0}{4} F_{ \mu\nu}F^{ \mu\nu} -$

$~-{\frac {1}{c}}U_{\mu }J^{\mu }-{\frac {c}{16\pi \eta }}u_{{\mu \nu }}u^{{\mu \nu }}-{\frac {1}{c}}\pi _{\mu }J^{\mu }-{\frac {c}{16\pi \sigma }}f_{{\mu \nu }}f^{{\mu \nu }}-{\frac {1}{c}}\lambda _{\mu }J^{\mu }-{\frac {c}{16\pi \tau }}h_{{\mu \nu }}h^{{\mu \nu }}){\sqrt {-g}}d\Sigma ,$

where is Lagrangian, is differential of coordinate time, is a certain coefficient, is the scalar curvature, $~\Lambda$ is the cosmological constant, which is a function of the system, is the speed of light as a measure of the propagation speed of electromagnetic and gravitational interactions, $~ D_\mu$ is the gravitational four-potential, is the gravitational constant, $~ \Phi_{ \mu\nu}$ is the gravitational tensor, $~ A_\mu$ is the electromagnetic 4-potential, $~ j^\mu$ is the electromagnetic (charge) 4-current, $~\varepsilon_0$ is the electric constant, $~ F_{ \mu\nu }$ is the electromagnetic tensor, $~U_{\mu }$ is the 4-potential of acceleration field, $~ \eta$ , $~ \sigma$ and $~ \tau$ are the constants of acceleration field, pressure field and dissipation field, respectively, $~ u_{ \mu\nu}$ is the acceleration tensor, $~ \pi_\mu$ is the 4-potential of pressure field, $~ f_{ \mu\nu}$ is the pressure field tensor, $~ \lambda_\mu$ is the 4-potential of dissipation field, $~ h_{ \mu\nu}$ is the dissipation field tensor, $~\sqrt {-g}d\Sigma= \sqrt {-g} c dt dx^1 dx^2 dx^3$ is the invariant 4-volume, $~\sqrt {-g}$ is the square root of the determinant of metric tensor, taken with a negative sign, is the product of differentials of the spatial coordinates.

The variation of the action function by 4-coordinates leads to the equation of motion of the matter unit in gravitational and electromagnetic fields, pressure field and dissipation field:^[3]

$-u_{{\beta \sigma }}\rho _{{0}}u^{\sigma }=\rho _{0}{\frac {dU_{\beta }}{d\tau }}-\rho _{0}u^{\sigma }\partial _{\beta }U_{\sigma }=\Phi _{{\beta \sigma }}\rho _{0}u^{\sigma }+F_{{\beta \sigma }}\rho _{{0q}}u^{\sigma }+f_{{\beta \sigma }}\rho _{0}u^{\sigma }+h_{{\beta \sigma }}\rho _{0}u^{\sigma },$

where the first term on the right is the gravitational force density, expressed with the help of the gravitational field tensor, second term is the Lorentz electromagnetic force density for the charge density $~ \rho_{0q}$ measured in the comoving reference frame, and the two last term set the pressure force density and the dissipation force density, respectively.

If we vary the action function by the dissipation 4-potential, we obtain the equation of dissipation field (3).

Dissipation stress-energy tensor

With the help of dissipation field tensor in the covariant theory of gravitation the dissipation stress-energy tensor is constructed:

$~ Q^{ik} = \frac{c^2} {4 \pi \tau }\left( -g^{im} h_{n m} h^{n k}+ \frac{1} {4} g^{ik} h_{m r} h^{m r}\right)$ .

The covariant derivative of the dissipation stress-energy tensor determines the dissipation four-force density:

$~f^{\alpha }=-\nabla _{\beta }Q^{{\alpha \beta }}={h^{\alpha }}_{{k}}J^{k}.$

Generalized velocity and Hamiltonian

Covariant 4-vector of generalized velocity is given by:

$~s_{{\mu }}=U_{{\mu }}+D_{{\mu }}+{\frac {\rho _{{0q}}}{\rho _{0}}}A_{{\mu }}+\pi _{{\mu }}+\lambda _{{\mu }}.$

With regard to the generalized 4-velocity, the Hamiltonian contains the dissipation field tensor and has the form:

$~H = \int {( s_0 J^0 - \frac {c^2}{16 \pi G} \Phi_{ \mu\nu}\Phi^{ \mu\nu}+ \frac {c^2 \varepsilon_0}{4} F_{ \mu\nu}F^{ \mu\nu }+ \frac {c^2 }{16 \pi \eta } u_{ \mu\nu} u^{ \mu\nu} + \frac {c^2 }{16 \pi \sigma } f_{ \mu\nu} f^{ \mu\nu}+ \frac {c^2 }{16 \pi \tau } h_{ \mu\nu} h^{ \mu\nu} ) \sqrt {-g} dx^1 dx^2 dx^3},$

where and are timelike components of 4-vectors $~ s_{\mu }$ and $~ J^{\mu }$ .

In the reference frame that is fixed relative to the center of mass of system, the Hamiltonian will determine the invariant energy of the system.

References

^1.0 ^1.1 Fedosin S.G. Four-Dimensional Equation of Motion for Viscous Compressible and Charged Fluid with Regard to the Acceleration Field, Pressure Field and Dissipation Field. International Journal of Thermodynamics. Vol. 18 (No. 1), pp. 13-24, 2015. http://dx.doi.org/10.5541/ijot.5000034003.
Fedosin S.G. About the cosmological constant, acceleration field, pressure field and energy. Jordan Journal of Physics. Vol. 9 (No. 1), pp. 1-30 (2016). http://dx.doi.org/10.5281/zenodo.889304.
Fedosin S.G. Equations of Motion in the Theory of Relativistic Vector Fields. International Letters of Chemistry, Physics and Astronomy, Vol. 83, pp. 12-30 (2019). https://doi.org/10.18052/www.scipress.com/ILCPA.83.12.

На русском языке