Relativistic energy and mass in the weak field limit

Jordan Journal of Physics. Vol. 8 (No. 1), pp. 1-16 (2015). http://journals.yu.edu.jo/jjp/Vol8No1Contents2015.html

Relativistic energy and mass in the weak field limit

Sergey G. Fedosin

PO box 614088, Sviazeva str. 22-79, Perm, Russia

Within the framework of the covariant theory of gravitation (CTG) the energy is calculated for a system with continuously distributed matter, taking into account the contribution of the gravitational and electromagnetic fields and the contribution of the pressure and acceleration fields. The total energy of all the fields is equal to zero, and the system’s energy is formed from the energy of the particles, which are under the influence of these fields. From the expression for the energy the inertial M and gravitational m_g masses of the system are found. These masses are compared with mass m_b, obtained by integrating the density over the volume, and with the total mass m’ of the body particles scattered to infinity in order to make the energy of macroscopic fundamental fields equal to zero. The ratio for the masses is obtained: m’ = M < m_b = m_g. From this the possibility of non-radiative ideal spherical collapse follows, when the system’s mass M does not change during the collapse. In addition, the mass of the system is less than the gravitational mass. In contrast, in the general theory of relativity (GTR) the ratio for masses is obtained in a different form: M = m_g < m_b < m’. In CTG the electromagnetic field energy reduces the gravitational mass and in GTR, on the contrary, increases. In order to verify the obtained results it is suggested to conduct an experiment on measuring the change of the gravitational mass of the body with increasing its electrical charge.

Keywords: relativistic energy; mass; acceleration field; pressure field; covariant theory of gravitation

1. Introduction

Modern physical theories usually describe the energy, momentum and mass of the system in four-dimensional formalism and introduce various 4-vectors and 4-tensors into consideration. In order to simplify comparison of the obtained expressions it is convenient to turn to such a weak field limit, that most of the formulas could be written in the same form as in the special theory of relativity, without loss of accuracy. In this work it will be done for the covariant theory of gravitation and general theory of relativity; particular attention will be paid to the meaning acquired by the mass in these theories.

2. Energy and mass in the covariant theory of gravitation

We will calculate the relativistic energy for the body in the form of a sphere with the uniform density of mass and charge, moving at velocity along the axis of the reference frame . The body under consideration is a set of identical particles moving randomly in different directions within the specified sphere with the radius . We will assume that all of these particles are held together by the force of gravitation. In order to simplify we will assume that the spaces between the particles are so small that integration over the volume of all the particles is equivalent to integration over the volume of the sphere. The sphere is at rest in the co-moving reference frame , associated with the center of mass, and the velocities of particles in are equal to and depend on the coordinates.

The Hamiltonian for continuously distributed matter in the covariant theory of gravitation is obtained from the Lagrangian with the help of Legendre transformations. This Hamiltonian is equal to the relativistic energy of the system and has the form [1-2]:

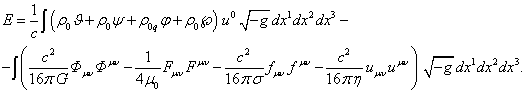(1)

Here is the speed of light, is the mass density of an arbitrary point particle in the reference frame associated with the particle, is the scalar potential of the acceleration field, is the scalar potential of the gravitational field, is the charge density in the reference frame , is the scalar potential of the electromagnetic field, is the scalar potential of the pressure field, denotes the timelike component of the 4-velocity of the particle, includes the determinant of the metric tensor with the minus sign, is an element of the three-dimensional volume in the reference frame , is the gravitational constant, is the gravitational tensor, is the vacuum permeability, is the electromagnetic tensor, is the acceleration tensor, is the pressure field tensor, and are constants.

For our purposes, it suffices to consider the expression for relativistic energy (1) in the case when the sphere under consideration is at rest in . Then all the calculations can be performed in the reference frame associated with the system’s center of mass. Let us assume that the gravitational field is small and the covariant theory of gravitation turns into the Lorentz-invariant theory of gravitation. In this case, the metric tensor no longer depends on the coordinates and is transformed into the metric tensor of Minkowski spacetime which is used in the special theory of relativity. For the case of the single fixed system the expressions for physical quantities are as follows:

, , , (2)

, , , .

where the Lorentz factor is ,

is the particle’s velocity in , is the gravitational field strength, is the torsion field vector, is the electric field strength, is the magnetic field induction, is the vacuum permittivity, is the pressure field strength, is the solenoidal vector of the pressure field, is the acceleration field strength, is the solenoidal vector of the acceleration field.

Substituting expressions (2) into (1) gives the following:

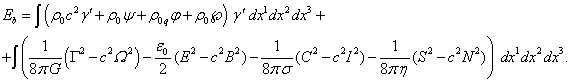(3)

First we will calculate the first integral in (3). According to [2], the Lorentz factor for the particles inside the fixed sphere is the function of the current radius :

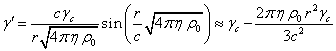, (4)

where is the Lorentz factor for velocities of the particles in the center of the sphere, and due to the smallness of the argument the sine can be expanded to the second-order terms.

For the first term in the first integral in (3) with regard to (4) in spherical coordinates we can write:

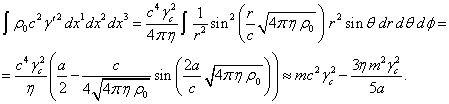(5)

In (5) the mass is the product of the density of the particles’ mass by the volume of the sphere which is at rest in the reference frame . The origin of the factor in (5) can be understood from the following. The quantity is the mass density of the particles in the center, which can be seen in the reference frame . Then the product gives the mass of the particles in the sphere for the observer in in the case, as if all the particles were in the center of the sphere. It is obvious that . In (5) it occurs that , that means that the total energy of the particles, increased due to the internal motion of the particles, is regarded by the Lorentz factor . The second term in (5) appears due to the radial gradient of mean velocities of the particles inside the sphere and it takes into account that not all the particles are located in the center of the sphere.

The scalar potential of the gravitational field in (3) inside the sphere, according to [2] is equal to:

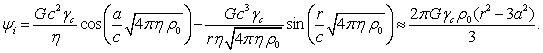(6)

Based on the similarity of the gravitational and electromagnetic fields, we can write for the electric potential similarly to (6):

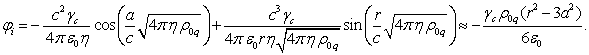
(7)

The scalar potential of the pressure field inside the sphere equals:

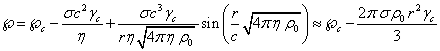, (8)

where denotes the potential of the pressure field in the center of the sphere.

Substituting (6), (7) and (8) in (3), taking into account (4) we find:

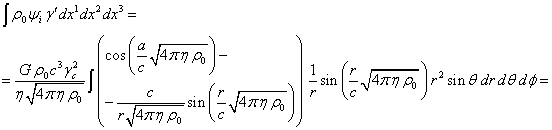
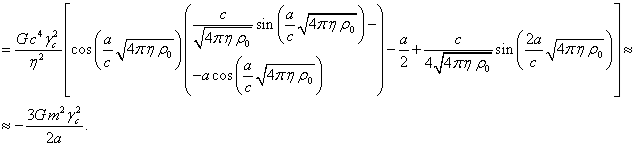(9)

(10)

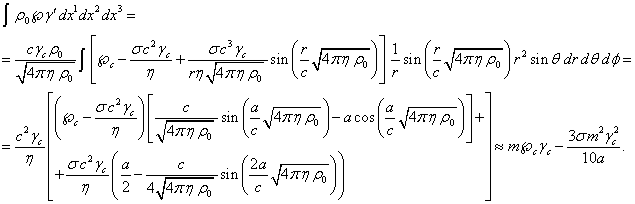
(11)

With regard to (5) and (9-11) the first integral in (3) will equal:

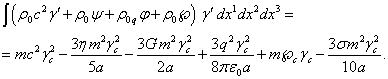(12)

The gravitational field strength and the torsion field inside the sphere are given by the formulas:

, , (13)

where is the vector potential of the gravitational field.

The vector potential of each particle is directed along its velocity, and due to random directions of the particles’ velocities the total vector potential inside and outside the sphere is zero. Consequently, the torsion field will also be zero: . Substituting the scalar potential (6) in (13), we find the gravitational field strength:

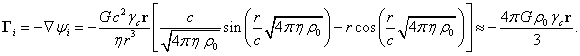
(14)

Taking into account (14) and the equality for the integral of the first term in the second integral in (3) we have:

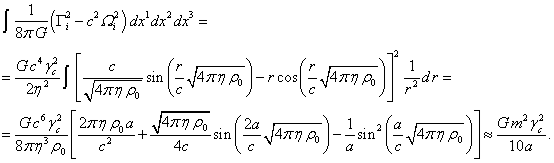(15)

According to [2], the potential of the gravitational field outside the sphere equals:

From this it follows that the gravitational mass of the sphere is equal to the quantity .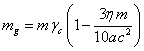

Using (13), with we find the field strength:

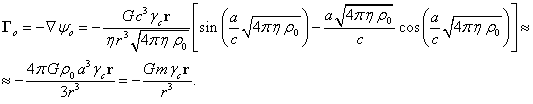
Substituting in (3), using the equation , we find for the gravitational field outside the sphere:

(16)

The sum of (15) and (16) equals:

. (17)

The calculation of the term with the electromagnetic field in (3) is done similarly and it gives for uniformly charged particles inside the stationary sphere the following:

, (18)

where the charge is the product of the charge density of an arbitrary particle in the reference frame associated with the particle by the volume of the stationary sphere.

In Minkowski space, the 4-velocity of the stationary sphere is , and based on the definition of the total 4-potential of the sphere’s pressure field , we find , , where denotes the density inside the stationary sphere. In this case the scalar potential , density and pressure inside the sphere are the functions of the current radius inside the sphere, and the equality for the vector potential of the pressure field in this case follows from the absence of ordered motion of particles inside the sphere. In view of this and (8) for , the vectors and inside the sphere are expressed as follows:

, .

In case of uniform mass density , calculations for the vector of the pressure strength inside the sphere give the following:

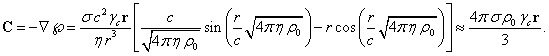
Using it we calculate the integral for the pressure:

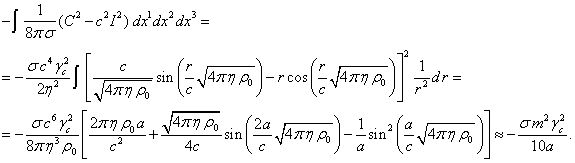
(19)

We have to calculate one more term in the second integral in (3). The components of vectors and for the acceleration field are found as follows:

, ,

where the scalar potential and the vector potential are part of the 4-potential of the acceleration field , which is a covariant 4-velocity.

In the limit of special theory of relativity , where is the Lorentz factor for the velocity of the particle’s motion. In the reference frame the particle’s velocities inside the sphere are equal to and should be used instead of . Then the potentials of an arbitrary particle will be , . We need the total potentials of the acceleration field inside the sphere, emerging due to direct interaction of the particles with each other and due to the influence of fields. In case of random motion of particles the velocities are directed in different directions, and therefore inside the sphere and . However, the total Lorentz factor of particles is a function of the current radius, and the total scalar potential is not equal to zero. With regard to (4), for it gives the following:

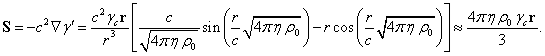
We will calculate the last integral:

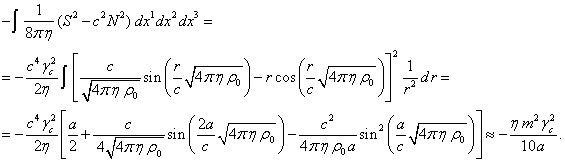
(20)

Substituting (12), (17), (18), (19) and (20) in (3), we find the relativistic energy of the system:

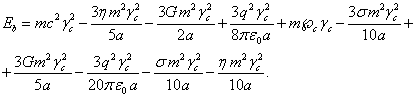(21)

In [2] the coefficients and were calculated for the case under consideration:

. (22)

If we substitute (22) in (21), we will see that the field energies are canceled completely. Only the energy of particles in corresponding fields remains:

. (23)

Equation (22) fixes a definite relation between the pressure field, acceleration field and gravitational and electromagnetic fields. This relation according to [2] reveals in the fact, that the conserved integral 4-vector, which is the result of integrating the equations of motion, is equal to zero. In this case condition (22) appears, and within the given model the 4/3 problem is explained.

Let us estimate the total mass of particles in the sphere, for which, taking into account (4), we integrate the mass density of particles in over the sphere’s volume:

(24)

Hence, by solving the quadratic equation we obtain: . Similarly we can link the charge with the charge of the sphere, which is found by the observer in : . We will substitute this in (23), given from (22):

. (25)

From (8) we will express the scalar potential of the pressure field in the center in terms of the potential near the surface of the sphere, and will consider the ratio :

. (26)

Similarly, from (4) we will express in terms of the Lorentz factor of the particles near the surface of the sphere:

. (27)

If we take into account (27) and (22) in (24), we can specify the relation between and :

. (28)

Substitution of (22), (26) and (27) in (25) gives the following:

. (29)

(29) shows that when the covariant theory of gravitation in the weak field limit turns into Lorentz- invariant theory of gravitation, all fields in the system, including the acceleration field, pressure field, electromagnetic and gravitational fields compensate each other so that the relativistic energy depends only on the mass, the energy of gravitational and electromagnetic fields, the energy of the surface pressure and the velocity of particles on the surface.

The scalar potential of the pressure field near the sphere’s surface is connected with the pressure by relation: , where and denote the pressure and the mass density near the surface of the sphere. Using the relation , where is the average density with respect to the sphere’s volume, we find: . For those massive bodies, in which we can assume and neglect the pressure on the surface, (29) becomes a simple expression:

. (30)

From (29) we will express the mass of the system, consisting of the matter mass and the mass of the four fields associated with this system:

. (31)

The mass is identical and at rest and in motion, and it is the invariant inertial mass of the system. Above we found from the formula for the external gravitational potential that the gravitational mass of the sphere is the quantity . Comparison with (24) shows that the sphere’s mass according to our assumptions is equal to the gravitational mass . According to (31), the system’s inertial mass increases relative to the mass by the value of mass-energy of the surface pressure, and to a certain share of the mass-energy of the electromagnetic field, but it decreases due to the same share of the mass-energy of the gravitational field.

3. Relations between the energies

We will compare the different energy components that make up the total relativistic energy (29). We will denote by , , and the energy components of the electromagnetic and gravitational fields, the pressure field and the acceleration field, respectively. As the measurement unit of energy we will use the sum of the energy components of the electromagnetic and gravitational fields from (17) and (18). Taking into account (24), (19), (20) and (22) we find:

. (32)

, .

According to (32), the energy components of the pressure field and acceleration field are twice less than the sum of the energy components of the gravitational and electromagnetic fields, and have a different sign. As a result, the sum of field energy components in (21) is equal to zero.

We will now consider the energy components of the matter particles which are under the influence of fields. We will denote these components by , , and , as the energy components of the particle in the electromagnetic and gravitational fields, the pressure field and the acceleration field, respectively. According to (9), (10), (11), (26), (5) and (27) we have the following:

, ,

, (33)

Now we will sum up the energy components in (32) and (33) separately for each field:

, ,

. (34)

The quantity denotes the sum of the energy components of the gravitational and electromagnetic fields, including the energy components of the fields themselves and of particles in these fields, the definition of is given in (32). The sum of all the energy components in (34) equals the relativistic energy of the system (29):

. (35)

If in (35) we neglect the product due to the small pressure on the body surface and disregard the rest energy , then the energy value remains, which is equal to: . In classical mechanics, in which the rest energy is not considered, the total energy of the gravitational and electromagnetic fields for a sphere with uniform distribution of mass and charge is equal to:

According to the virial theorem, it is considered that the internal kinetic energy should equal half the absolute value of the energy of fields: . The total energy is composed of the energy of fields and the internal energy:

. (36)

This shows that the total energy in classical mechanics coincide with the relativistic energy (35), if we exclude from the latter the rest energy and the energy of the surface pressure. Thus the transition is performed of the covariant theory of gravitation into the classical mechanics. However, in classical mechanics it is not determined how the internal pressure makes contribution to the mass and energy of the system.

We will now specify, how in our model the virial theorem is realized, particularly for field energies and particle energies. We have the energy of the pressure field and the energy of the acceleration field, and the sum of these energies, according to (32), is equal to the absolute value of the sum of energies of the gravitational and electromagnetic fields. As a result, the sum of fields’ energies is equal to zero.

The situation for the energies of particles in fields is different. The energy of a particle in the field in the absence of the vector potential is defined by the product of the mass (charge) by the scalar potential. The sum of the energies of particles in the gravitational and electromagnetic fields according to (33) is equal to , the energy of particles in the pressure field is , and the energy of particles in the acceleration field is . From the energy of particles in the pressure field we can distinguish the energy and the energy – from the energy of particles in the acceleration field. But the sum of these energies is times less than the absolute value of the sum of energies of particles in the gravitational and electromagnetic fields. At the same time, the excess energy of particles in the gravitational and electromagnetic field, which is equal to , is compensated by the fact, that the gravitational mass energy of the system increases from to .

4. Relation with the cosmological constant

In [1] we obtained a relation that connects the cosmological constant with the 4-potentials of fields, which are included in the Lagrangian:

. (37)

Let us expand the products of 4- vectors:

, ,

here is the mass 4-current; is the charge (electromagnetic) 4-current; , , and denote the vector potentials of the acceleration field, gravitational and electromagnetic fields and pressure field, respectively; and we use the approximation of the special theory of relativity, in which , where , is the velocity of motion of the body’s arbitrary particle.

Let us consider the situation in the reference frame , which is stationary relative to the body in question. In the particle velocities are equal to and the Lorentz factor should be used instead of . As a result, (37) can be rewritten as follows:

. (38)

In relation (38) the cosmological constant has its own value for each particle of the body. We intend to integrate (38) over the volume of the body in the form of a fixed sphere, which is filled with moving particles as tightly as possible, and which has uniform density of mass and charge in the entire volume of the sphere. In the absence of general rotation or directed matter flows the particles’ velocities are directed randomly in different directions. Then, after integrating (38) the contribution of vector products containing will be zero, and the total vector potentials , , and inside the sphere will be zero as well. Therefore, the integral of (38) over the volume is as follows:

The quantity in our opinion is the energy density of each particle, and the integral of this density over the volume gives a certain energy constant , which is associated with all the particles of the system. In the right side of the equation there is the integral that we have already calculated in (12). With this in mind, we can write:

. (39)

If we compare (39) with (21), we see that the quantity is part of the relativistic energy of the system, and denotes the sum of energy components of the particles under the influence of fields. The energy also includes the energy components, associated with the fields themselves, but according to (23) in case of a spherical body all these components cancel each other. Therefore, we can assume that for a sphere , and .

In (39) the mass is some constant mass, which denotes the total mass of body particles, excluding the contribution from the mass-energy of macroscopic fields, associated with this body. If we divide the total body matter by particles and scatter from each other to infinity, then for the matter at rest there will be no electromagnetic and gravitational fields, associated with the interaction of these particles with each other. There will be no internal pressure from the particles’ influence on each other. In this case, with regard to (38) written for a single particle, the mass will consist of the total mass of all the particles in view of the energy of particles’ proper fields, the energy of their internal pressure and the internal kinetic energy. We considered such mass in [4] as the total mass of the body parts, scattered from each other and located at infinity at zero absolute temperature. At infinity , , and then the system’s mass turns into the mass .

From (29-30) it follows that the system mass is less than the body mass: , and the body mass is equal to the gravitational mass . Since the mass is constant and is associated with the cosmological constant, and , it turns out that the gravitational mass of the system in (29) can change, when in the system there is a change in the energy of the pressure field or the energy of the electromagnetic and gravitational fields. From (28) we find that , and is in the middle between and . As a result, the ratio of the masses is as follows:

. (40)

5. Discussion of results

5.1. The masses

According to (40) in the weak field the inertial mass of the system in the form of a sphere with particles, taking into account the field energies, the internal pressure and the internal kinetic energy can be described either by the formula (29) or by the system mass from (39). The equality means conservation of the system’s energy, regardless of whether the system’s parts are at infinity and do not interact with each other, or these parts come into close contact and form a coupled system. This is possible in case of ideal spherical collapse, when there are no emission and matter ejections from the system at any stage of the collapse or the matter accumulation. We discussed this question in [2] in connection with the problem of energy in spherical supernova collapse. There we explained the possibility of low energy emission by neutrinos based on the fact that almost all the work of the gravitational forces during the matter compression can come on increasing the kinetic energy of the stellar matter motion and the pressure energy, as well as on creating the internal pressure gradients and particle’s velocities.

Earlier in [5] we found the expression for the masses, which differs from (40): . We can explain this by a different accepted gauge of the cosmological constant – in this paper we use the formulas obtained with the gauge according to [1], which differs from the gauge in [5]. Also, we are currently using for analysis another physical system in the form of a sphere, consisting of a set of particles moving inside the sphere, which are held together by gravitation. In such a system inevitably there is difference between the masses and , as a consequence of the radial gradient of the Lorentz factor inside the sphere and as a consequence of the difference between the density of the particles in the reference frame and the density of particles from the standpoint of the reference frame , associated with the system’s center of mass. The mass in (40) in its meaning has technical nature, since it is determined only mathematically by multiplying the density by the sphere’s volume. We will note that the density is included in the system’s Lagrangian with the 4-vector of the gravitational (mass) current density in the form . The density is also included in the equation of motion of a point particle and in the field equations in [1].

According to (29) and (40), the mass is greater than the mass . This means that the gravitational mass of the system is always greater than the inertial mass of the system, by the half the absolute value of the gravitational and electromagnetic field energy minus the mass-energy of the surface pressure.

According to (40) the gravitational mass is also greater than the mass of the system’s parts, scattered to infinity. We can explain this in the following way. As we know, for a ball the absolute value of the potential energy of the gravitational field is equal to the total work on the matter transfer from infinity to the surface and inside the ball. It is assumed that the ball is formed by gradual growth due to layering of spherical shells as the matter is transferred. But beside the fact that the matter is transferred from infinity inside the body, which results in increase of the absolute value of the potential energy of the body’s gravitational field, the force of gravitation performs other actions – it increases the kinetic energy of the particles inside the body, the energy of the particles’ pressure on each other, and creates the gradients of pressure and kinetic energy of the particles inside the body. All these types of work of the gravitation force on the body formation increase the body mass from to . The main contribution to the gravitational mass increase is made by the emerging motion – at infinity the particles were stationary, but inside the body the particles move at velocities .

If we consider the virial theorem, connecting half the absolute value of gravitational and electromagnetic energies with the internal energy of the body, then it turns out that half of the work of the gravitational and electromagnetic fields on the body formation is transformed into the internal energy of the body. The total energy of the body, according to (36), is negative and with the help of it (35), (39) and (29) can be written as follows:

. (41)

Since is equal to half the sum of the gravitational and electrical energies, then we can see that half of the work of the gravitational and electromagnetic fields on the body formation is transformed into the mass increase from to the value .

From the virial theorem the approximate equality follows between the absolute value of the total system’s energy (36), the internal body energy and the binding energy, if we define it in (41) as the difference between the rest energy for the mass and the rest energy of the initial state at infinity . However, in usual interpretation of the binding energy it is not so, since the binding energy is defined as the difference between the total energy of the individual parts of the system and the energy of the system made up of these parts into a whole. This definition of the binding energy in this case gives us the relation: , i.e. in case of ideal spherical collapse the system’s energy at the beginning and the end of the process is the same and the binding energy is equal to zero. Despite the equality of the binding energy to zero, the system does not fall apart because the masses are always attracted. And the total energy (36) of the system remains negative.

The invariant mass of the system is the measure of inertia of the system as a whole and the measure of the relativistic energy of the system. This means that the system’s acceleration under the influence of forces should depend on the mass . The mass can be calculated as the integral of the density over the volume of the sphere. The gravitational mass is equal to and can be determined by means of gravitational experiments near the body on the gravitational effect on the test bodies. According to (31), at an infinitely large radius of the body the mass of the spherical system becomes equal to the gravitational mass of the body . Equation (31) can be regarded as the quadratic equation to determine the gravitational mass depending on the body radius , on its electrical charge and the total mass of the fixed parts of this body , when these parts are motionless and infinitely distant from each other:

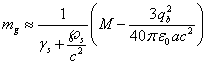5.2. Energies and masses in the general theory of relativity

In the general theory of relativity (GTR) the system’s mass is considered to be less than the total mass of the body’s parts [6-7]. In GTR, there is gravitational mass of the system from the standpoint of a distant observer, calculated as the volume integral of the sum , where is the concentration of matter nucleons, is the mass of one nucleon, is the density of the body’s internal mass-energy [8]. The inertial mass of the system is also considered, which is calculated with the volume integral of the timelike component of the stress-energy tensor, which is then divided by the square of the speed of light and equated to the gravitational mass based on the principle of equivalence. Accordingly, to determine the system’s mass we need to know either the internal energy of the body which is not precisely known, or use the stress-energy tensor, which however does not include the gravitational field energy in principle. The latter is due to the fact that in GTR the gravitational field is understood as a metric field and is described by the stress-energy pseudotensor. As a result, calculation of the relativistic energy and the system’s mass in GTR is much more difficult and involves a number of conditions. For example, for calculating the energy the coordinates of the reference frame at infinity should transfer into the coordinates of Minkowski space.

The mass of the system, with regard of the gravitational and electromagnetic fields, according to [6] and [9], in GTR in the weak field in our notation relative to the mass, density and radius of the body is equal to:

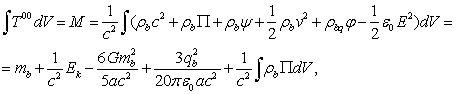(42)

where is the mass tensor, turning after multiplying by the square of the speed of light into the stress-energy tensor of the system; the body mass ; and are the density of mass and charge, respectively; is the kinetic energy; is the pressure energy per unit mass, and the case of uniform density is considered.

In [6] also the invariant mass density is used, which implies such mass density, which does not change under the influence of the pressure or gravitational field. It is assumed that such invariant density is part of the continuity relation in the curved spacetime: , here is the determinant of the metric tensor, is the 4-velocity. We will note in this regard, that in the covariant theory of gravitation the continuity relation is written not for but for [1], and can vary and depend on any factors, including the pressure and gravitational field.

In the weak field for the fixed body in GTR may be written:

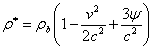. (43)

We will assume that , as it should be expected due to virial theorem. If we substitute (43) into (42), we obtain the relation: , so that the mass of the system is greater than the mass . After substituting (43) into (42), we obtain the expression for the mass-energy of the system, which to similar to those presented in [7] and [10] (in contrast to [6], in [10] is an invariant density and denotes the mass density corresponding to our density ).

We will assume that the mass of the system in (42) according to GTR is calculated precisely and is equal to our mass of the system in (31):

(44)

From the left side of (44) we see that in GTR the gravitational energy is included in the equation with the increased weight relative to the electromagnetic energy, and in the right side both energies have the same weight due to the similarity of equations for the fields. This is due to the fact that in GTR the gravitational field is replaced by the effect of the action of the metric field of the metric tensor. As a result, the entire metric contains gravitation and the electromagnetic field and pressure remain independent.

If we neglect the contribution of to (44) and consider this quantity as a unity, then with regard to the expression from (44) we can estimate the pressure energy in GTR:

In (42) the mass of the system due to the equivalence principle is considered equal to the gravitational mass. This means that in GTR a charged body increases its gravitational mass. Based on the stated above, the ratio of masses in GTR is as follows:

, (45)

where in the first approximation (here is the number of nucleons in the body, is the mass of a nucleon), ( is the mass of the system in the form of the body and its fields, is the internal energy in (36) ), the mass is equal to the gravitational mass , the mass is determined by the integral over the volume of the invariant density (43), the mass is calculated by integrating over the volume of the body density , and the mass is determined by us in (28) with the help of and has technical nature.

If the mass of the system decreases from the value to , then there is excess energy of the order of . In GTR the collapsing system must radiate this energy, so that the ideal spherical non-radiating collapse in GTR is impossible [8].

As we can see, relation (45) for the masses in GTR differs significantly from relation (40) for the masses in the covariant theory of gravitation.

6. Conclusion

According to (32), the total energy of the gravitational and electromagnetic fields summed up with the energy of the acceleration field and the energy of the pressure field inside the spherical body is equal to zero. During the body formation distribution of energies of the body particles takes place in the potentials of all the four fields. This leads to the kinetic energy of the motion of particles, to the internal pressure and the energy of particles in the gravitational and electromagnetic fields.

The difference of our approach from the results of GTR is that the mass of the system in the ideal spherical collapse does not change, . Really, if at the beginning of the ideal collapse the spatial component of the total 4-momentum of the particles falling on the center of mass is equal to zero due to the spherical symmetry, the same will take place at the end of the collapse, so that the mass-energy, which is part of the time component of 4-momentum, may be conserved. However, the gravitational mass is greater than the mass of the system, since the state of the particles changes – they start moving inside the system and exert pressure on each other, besides, the particles acquire additional energy in the internal fields.

If the system contains the electromagnetic field, its influence on the mass is opposite to the influence of the gravitational field, i.e. the electromagnetic field must reduce the gravitational mass . We can calculate, that if a body with the mass of 1 kg and the radius of 1 meter is charged up to the potential of about 5 megavolt, it must reduce the gravitational mass of the body (not including the mass of the additional charges) at weighing in the gravity field by mass fraction, which is close to the present day accuracy of mass measurement.

7. References

1. Fedosin S.G. About the cosmological constant, acceleration field, pressure field and energy. vixra.org, 5 Mar 2014.

2. Fedosin S.G. The Integral Energy-Momentum 4-Vector and Analysis of 4/3 Problem Based on the Pressure Field and Acceleration Field. American Journal of Modern Physics. Vol. 3, No. 4, 2014, pp. 152-167. doi: 10.11648/j.ajmp.20140304.12.

3. Fedosin S.G. 4/3 Problem for the Gravitational Field. Advances in Physics Theories and Applications, 2013, Vol. 23, P. 19 – 25.

4. Fedosin S.G. Energy, Momentum, Mass and Velocity of a Moving Body in the Light of Gravitomagnetic Theory. Canadian Journal of Physics, 2014, Vol. 92, no. 10, P. 1074 – 1081. http://dx.doi.org/10.1139/cjp-2013-0683.

5. Fedosin S.G. The Hamiltonian in Covariant Theory of Gravitation. Advances in Natural Science, 2012, Vol. 5, No. 4, P. 55 – 75.

6. Fock V. A. The Theory of Space, Time and Gravitation (Pergamon Press, London, 1959).

7. Papapetrou A. Equations of Motion in General Relativity. Proc. Phys. Soc. A, 64 (1951), P. 57.

8. Abhas Mitra. Why Gravitational Contraction Must be Accompanied by Emission of Radiation both in Newtonian and Einstein Gravity. Phys. Rev. D Vol. 74, 024010 (2006).

9. Landau L.D. and Lifshitz E.M. The Classical Theory of Fields (Vol. 2, 4th ed. Butterworth-Heinemann, 1975).

10. Chandrasekhar S. The Post-Newtonian Equations of Hydrodynamics in General Relativity. Ap. J. 142 (1965), P. 1488; Ap. J. 158 (1969), P. 45.

Source: http://sergf.ru/reen.htm

Scientific site